2C4H6(g) → C8H12(g) 500 K 1.4×10^(-2) M-1 s-1 0.020 M

2C4H6(g) → C8H12(g) 500 K 1.4×10^(-2) M-1 s-1 0.020 M

2C4H6(g) → C8H12(g) 반응에 대해서

500 K에서 속도 상수는 1.4×10^(-2) M-1 s-1이며,

C4H6(g)의 처음 농도는 0.020 M이다.

a) 반응 차수

b) 반감기

c) 같은 온도에서 1시간 후 남아있는 C4H6(g)의 농도

For the reaction 2C4H6(g) → C8H12(g), the rate constant at 500 K is 1.4×10^(-2) M-1 s-1, and the initial concentration of C4H6(g) is 0.020 M.

a) 반응 차수

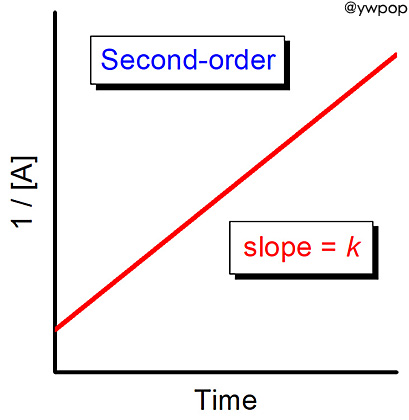
속도 상수의 단위가 M-1 s-1이므로,

2차 반응

( 참고 https://ywpop.tistory.com/25 )

b) 반감기

2차 반응의 반감기

t_1/2 = 1 / (k [A]_0)

= 1 / [(1.4×10^(-2) M-1 s-1) (0.020 M)]

= 1 / [(1.4×10^(-2)) (0.020)]

= 3571.43 s

= 3.6×10^3 s

c) 같은 온도에서 1시간 후 남아있는 C4H6(g)의 농도

1시간 = 3600초 이고,

3600초는 반감기와 유사한 값이므로,

1시간 후 남아있는 C4H6(g)의 농도는

0.020 M의 1/2인 0.010 M일 것이다.

2차 반응 속도식

(1 / C_t) = k•t + (1 / C_0)

C_t = 1 / [k•t + (1 / C_0)]

= 1 / [(1.4×10^(-2))×(3600) + (1 / 0.020)]

= 0.00996 M

= 0.010 M

[키워드] 2차 반응 기준, 반응 속도론

[구글에 색인된 페이지]

In the gas phase, two butadiene molecules can dimerizes to given larger molecules according to the reaction: 2C4H6(g) → C8H12(g).

The reaction of butadiene gas (C4H6) with itself produces C8H12 gas as follows: 2C4H6(g) → C8H12(g). The reaction is second-order, with a rate constant of 5.76×10^(-2) M-1 min-1. If the initial concentration of butadiene is 0.200 M, what concentration will remain after 10.0 min?